Can the Great Circle for Navigation Be Shown Using Drawings

Flight or sailing route along the shortest path between two points on a globe's surface

Orthodromic course drawn on the earth world.

Great-circle navigation or orthodromic navigation (related to orthodromic course; from the Greek ορθóς, right angle, and δρóμος, path) is the do of navigating a vessel (a transport or aircraft) forth a bang-up circle. Such routes yield the shortest altitude between two points on the world.^[1]

Form [edit]

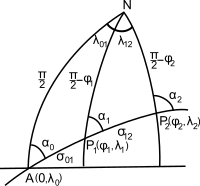
Effigy 1. The corking circle path betwixt (φ₁, λ₁) and (φ_two, λ₂).

The not bad circle path may exist found using spherical trigonometry; this is the spherical version of the changed geodetic trouble. If a navigator begins at P ₁ = (φ_ane,λ₁) and plans to travel the corking circle to a point at betoken P ₂ = (φ₂,λ_ii) (meet Fig. ane, φ is the latitude, positive northward, and λ is the longitude, positive eastward), the initial and concluding courses α₁ and α₂ are given by formulas for solving a spherical triangle

${\displaystyle {\begin{aligned}\tan \blastoff _{1}&={\frac {\cos \phi _{2}\sin \lambda _{12}}{\cos \phi _{i}\sin \phi _{2}-\sin \phi _{1}\cos \phi _{2}\cos \lambda _{12}}},\\\tan \blastoff _{2}&={\frac {\cos \phi _{i}\sin \lambda _{12}}{-\cos \phi _{2}\sin \phi _{i}+\sin \phi _{2}\cos \phi _{one}\cos \lambda _{12}}},\\\end{aligned}}}$

where λ₁₂ = λ₂ − λ₁ ^{[note one]} and the quadrants of α_one,α₂ are determined by the signs of the numerator and denominator in the tangent formulas (eastward.g., using the atan2 function). The central bending between the two points, σ₁₂, is given by

${\displaystyle \tan \sigma _{12}={\frac {\sqrt {(\cos \phi _{1}\sin \phi _{ii}-\sin \phi _{one}\cos \phi _{2}\cos \lambda _{12})^{ii}+(\cos \phi _{ii}\sin \lambda _{12})^{2}}}{\sin \phi _{1}\sin \phi _{2}+\cos \phi _{ane}\cos \phi _{2}\cos \lambda _{12}}}.}$ ^{[note two] [note three]}

(The numerator of this formula contains the quantities that were used to determine tanα₁.) The distance forth the great circumvolve will and so exist s ₁₂ =Rσ₁₂, where R is the assumed radius of the earth and σ₁₂ is expressed in radians. Using the mean world radius, R =R ₁ ≈ 6,371 km (3,959 mi) yields results for the distance s ₁₂ which are within 1% of the geodesic length for the WGS84 ellipsoid; meet Geodesics on an ellipsoid for details.

Finding manner-points [edit]

To find the way-points, that is the positions of selected points on the nifty circle betwixt P ₁ and P ₂, we first extrapolate the great circumvolve back to its node A, the signal at which the bang-up circle crosses the equator in the n direction: let the longitude of this bespeak be λ₀ — encounter Fig i. The azimuth at this indicate, α₀, is given past

${\displaystyle \tan \alpha _{0}={\frac {\sin \alpha _{one}\cos \phi _{1}}{\sqrt {\cos ^{ii}\alpha _{1}+\sin ^{two}\blastoff _{1}\sin ^{2}\phi _{1}}}}.}$ ^{[note four]}

Let the angular distances along the great circumvolve from A to P _i and P ₂ exist σ₀₁ and σ₀₂ respectively. Then using Napier'south rules nosotros have

${\displaystyle \tan \sigma _{01}={\frac {\tan \phi _{ane}}{\cos \alpha _{i}}}\qquad }$ (If φ₁ = 0 and α_ane = ane⁄2 π, apply σ₀₁ = 0).

This gives σ₀₁, whence σ₀₂ = σ₀₁ + σ₁₂.

The longitude at the node is constitute from

${\displaystyle {\begin{aligned}\tan \lambda _{01}&={\frac {\sin \blastoff _{0}\sin \sigma _{01}}{\cos \sigma _{01}}},\\\lambda _{0}&=\lambda _{1}-\lambda _{01}.\cease{aligned}}}$

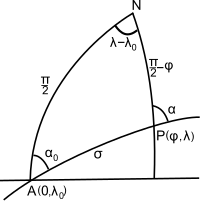
Figure 2. The great circumvolve path betwixt a node (an equator crossing) and an capricious betoken (φ,λ).

Finally, calculate the position and azimuth at an arbitrary bespeak, P (see Fig. ii), by the spherical version of the direct geodesic problem.^{[note v]} Napier'south rules requite

${\displaystyle {\color {white}.\,\qquad )}\tan \phi ={\frac {\cos \alpha _{0}\sin \sigma }{\sqrt {\cos ^{2}\sigma +\sin ^{2}\blastoff _{0}\sin ^{2}\sigma }}},}$ ^{[note 6]}

${\displaystyle {\brainstorm{aligned}\tan(\lambda -\lambda _{0})&={\frac {\sin \alpha _{0}\sin \sigma }{\cos \sigma }},\\\tan \alpha &={\frac {\tan \alpha _{0}}{\cos \sigma }}.\terminate{aligned}}}$

The atan2 function should be used to make up one's mind σ₀₁, λ, and α. For instance, to find the midpoint of the path, substitute σ = 1⁄2 (σ₀₁ + σ₀₂); alternatively to observe the signal a distance d from the starting bespeak, take σ = σ₀₁ +d/R. Likewise, the vertex, the signal on the bang-up circle with greatest breadth, is institute by substituting σ = + i⁄2 π. It may be convenient to parameterize the road in terms of the longitude using

${\displaystyle \tan \phi =\cot \alpha _{0}\sin(\lambda -\lambda _{0}).}$ ^{[notation 7]}

Latitudes at regular intervals of longitude tin can be constitute and the resulting positions transferred to the Mercator chart assuasive the dandy circle to be approximated by a series of rhumb lines. The path determined in this way gives the great ellipse joining the end points, provided the coordinates ${\displaystyle (\phi ,\lambda )}$ are interpreted as geographic coordinates on the ellipsoid.

These formulas utilize to a spherical model of the earth. They are also used in solving for the bang-up circle on the auxiliary sphere which is a device for finding the shortest path, or geodesic, on an ellipsoid of revolution; see the article on geodesics on an ellipsoid.

Example [edit]

Compute the swell circumvolve route from Valparaíso, φ_one = −33°, λ₁ = −71.vi°, to Shanghai, φ₂ = 31.4°, λ₂ = 121.8°.

The formulas for course and distance requite λ₁₂ = −166.6°,^{[note 8]} α₁ = −94.41°, α₂ = −78.42°, and σ₁₂ = 168.56°. Taking the earth radius to be R = 6371 km, the altitude is due south ₁₂ = 18743 km.

To compute points along the route, beginning find α₀ = −56.74°, σ₁ = −96.76°, σ₂ = 71.8°, λ₀₁ = 98.07°, and λ₀ = −169.67°. Then to compute the midpoint of the route (for instance), have σ = 1⁄2 (σ₁ + σ_ii) = −12.48°, and solve for φ = −half-dozen.81°, λ = −159.18°, and α = −57.36°.

If the geodesic is computed accurately on the WGS84 ellipsoid,^[iv] the results are α₁ = −94.82°, α₂ = −78.29°, and s ₁₂ = 18752 km. The midpoint of the geodesic is φ = −seven.07°, λ = −159.31°, α = −57.45°.

Gnomonic nautical chart [edit]

A direct line drawn on a gnomonic chart would be a great circle track. When this is transferred to a Mercator nautical chart, it becomes a curve. The positions are transferred at a user-friendly interval of longitude and this is plotted on the Mercator nautical chart.

Run across as well [edit]

• Compass rose
• Great circle
• Great-circumvolve distance
• Neat ellipse
• Geodesics on an ellipsoid
• Geographical distance
• Isoazimuthal
• Loxodromic navigation
• Map
  • Portolan map
• Marine sandglass
• Rhumb line
• Spherical trigonometry
• Windrose network

Notes [edit]

1. ^ In the article on dandy-circle distances, the note Δλ = λ₁₂ and Δσ = σ₁₂ is used. The note in this commodity is needed to deal with differences between other points, due east.k., λ₀₁.
2. ^ A simpler formula is

   ${\displaystyle \cos \sigma _{12}=\sin \phi _{1}\sin \phi _{2}+\cos \phi _{1}\cos \phi _{2}\cos \lambda _{12};}$

   however, this is less accurate if σ₁₂ small.
3. ^ These equations for α₁,α₂,σ₁₂ are suitable for implementation on modern calculators and computers. For hand computations with logarithms, Delambre's analogies^[ii] were usually used:

   ${\displaystyle {\begin{aligned}\cos {\tfrac {ane}{2}}(\alpha _{two}+\alpha _{1})\sin {\tfrac {i}{2}}\sigma _{12}&=\sin {\tfrac {1}{2}}(\phi _{ii}-\phi _{1})\cos {\tfrac {i}{two}}\lambda _{12},\\\sin {\tfrac {1}{2}}(\blastoff _{2}+\blastoff _{one})\sin {\tfrac {ane}{2}}\sigma _{12}&=\cos {\tfrac {1}{2}}(\phi _{two}+\phi _{1})\sin {\tfrac {one}{2}}\lambda _{12},\\\cos {\tfrac {1}{two}}(\alpha _{ii}-\alpha _{1})\cos {\tfrac {i}{2}}\sigma _{12}&=\cos {\tfrac {1}{ii}}(\phi _{2}-\phi _{1})\cos {\tfrac {1}{2}}\lambda _{12},\\\sin {\tfrac {i}{2}}(\alpha _{two}-\alpha _{i})\cos {\tfrac {1}{2}}\sigma _{12}&=\sin {\tfrac {one}{2}}(\phi _{2}+\phi _{1})\sin {\tfrac {1}{two}}\lambda _{12}.\end{aligned}}}$

   McCaw^[3] refers to these equations as existence in "logarithmic form", by which he means that all the trigonometric terms appear as products; this minimizes the number of table lookups required. Furthermore, the back-up in these formulas serves as a bank check in mitt calculations. If using these equations to determine the shorter path on the not bad circle, it is necessary to ensure that |λ₁₂| ≤ π (otherwise the longer path is found).
4. ^ A simpler formula is

   ${\displaystyle \sin \alpha _{0}=\sin \alpha _{1}\cos \phi _{one};}$

   however, this is less authentic α₀ ≈ ± 1⁄2 π.
5. ^ The straight geodesic problem, finding the position of P _ii given P _one, α₁, and s ₁₂, can also be solved by formulas for solving a spherical triangle, equally follows,

   ${\displaystyle {\brainstorm{aligned}\sigma _{12}&=s_{12}/R,\\\sin \phi _{2}&=\sin \phi _{1}\cos \sigma _{12}+\cos \phi _{1}\sin \sigma _{12}\cos \alpha _{i},\quad {\text{or}}\\\tan \phi _{two}&={\frac {\sin \phi _{ane}\cos \sigma _{12}+\cos \phi _{i}\sin \sigma _{12}\cos \blastoff _{1}}{\sqrt {(\cos \phi _{1}\cos \sigma _{12}-\sin \phi _{1}\sin \sigma _{12}\cos \alpha _{one})^{2}+(\sin \sigma _{12}\sin \blastoff _{1})^{two}}}},\\\tan \lambda _{12}&={\frac {\sin \sigma _{12}\sin \alpha _{1}}{\cos \phi _{i}\cos \sigma _{12}-\sin \phi _{1}\sin \sigma _{12}\cos \alpha _{1}}},\\\lambda _{2}&=\lambda _{1}+\lambda _{12},\\\tan \blastoff _{2}&={\frac {\sin \alpha _{one}}{\cos \sigma _{12}\cos \alpha _{i}-\tan \phi _{1}\sin \sigma _{12}}}.\stop{aligned}}}$

   The solution for way-points given in the main text is more general than this solution in that it allows mode-points at specified longitudes to be found. In addition, the solution for σ (i.e., the position of the node) is needed when finding geodesics on an ellipsoid past means of the auxiliary sphere.
6. ^ A simpler formula is

   ${\displaystyle \sin \phi =\cos \alpha _{0}\sin \sigma ;}$

   however, this is less authentic when φ ≈ ± ane⁄2 π
7. ^ The following is used: ${\displaystyle \cos \sigma =\cos \phi \cos(\lambda -\lambda _{0})}$
8. ^ λ₁₂ is reduced to the range [−180°, 180°] by adding or subtracting 360° equally necessary

References [edit]

1. ^ Adam Weintrit; Tomasz Neumann (vii June 2011). Methods and Algorithms in Navigation: Marine Navigation and Safety of Body of water Transportation. CRC Printing. pp. 139–. ISBN978-0-415-69114-7.
2. ^ Todhunter, I. (1871). Spherical Trigonometry (3rd ed.). MacMillan. p. 26.
3. ^ McCaw, Thou. T. (1932). "Long lines on the Earth". Empire Survey Review. i (6): 259–263. doi:10.1179/sre.1932.1.6.259.
4. ^ Karney, C. F. F. (2013). "Algorithms for geodesics". Periodical of Geodesy. 87 (one): 43–55. doi:ten.1007/s00190-012-0578-z.

External links [edit]

• Great Circumvolve – from MathWorld Swell Circle description, figures, and equations. Mathworld, Wolfram Research, Inc. c1999
• Great Circle Mapper Interactive tool for plotting bang-up circle routes.
• Neat Circle Computer deriving (initial) grade and distance between two points.
• Great Circumvolve Altitude Graphical tool for drawing great circles over maps. Also shows distance and azimuth in a table.
• Google assistance program for orthodromic navigation

welchvilven.blogspot.com

Source: https://en.wikipedia.org/wiki/Great-circle_navigation

Share this post